List of Trigonometry Formulas PDF
Çözemediğin YKS, LGS ve KPSS sorularının fotoğrafını çek, adım adım çözümü anında cebine gelsin!
Trigonometry formulas are a set of different formulas containing trigonometric identities used to solve problems based on the sides and angles of a right-angled triangle. In our article, we will list the trigonometry formulas. We will see trigonometric identities. You will also be able to download these formulas in PDF form.
List of Trigonometry Formulas
Basic Trigonometry Formulas
Trigonometric Ratio Formulas
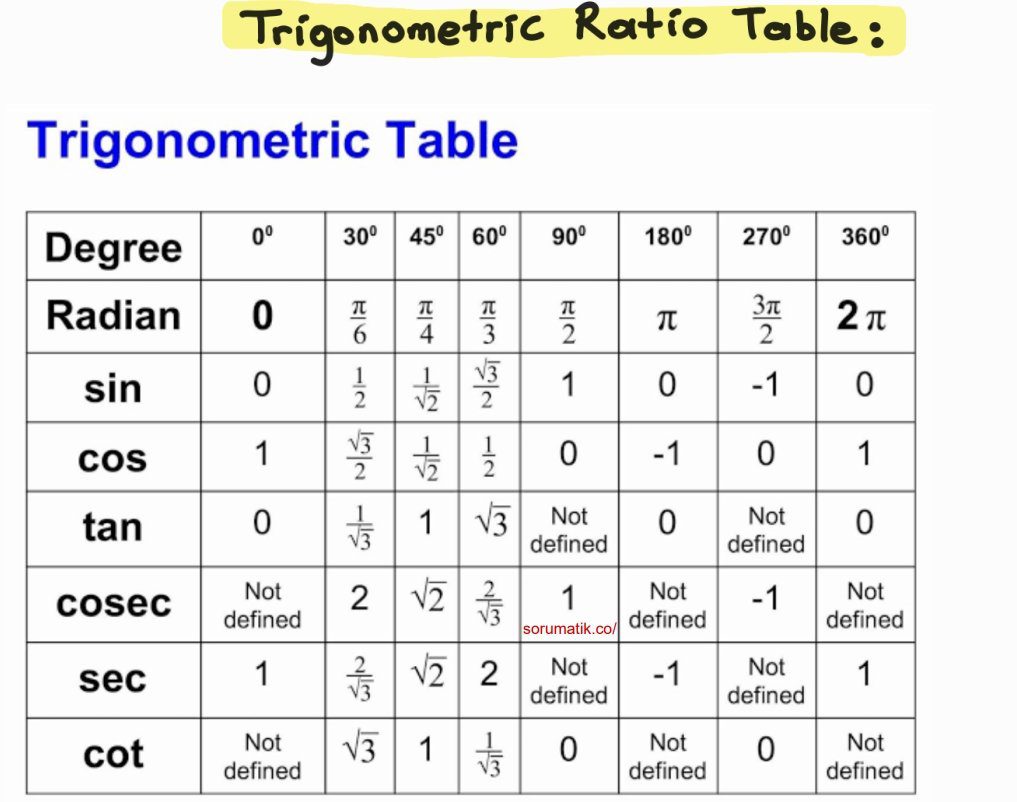
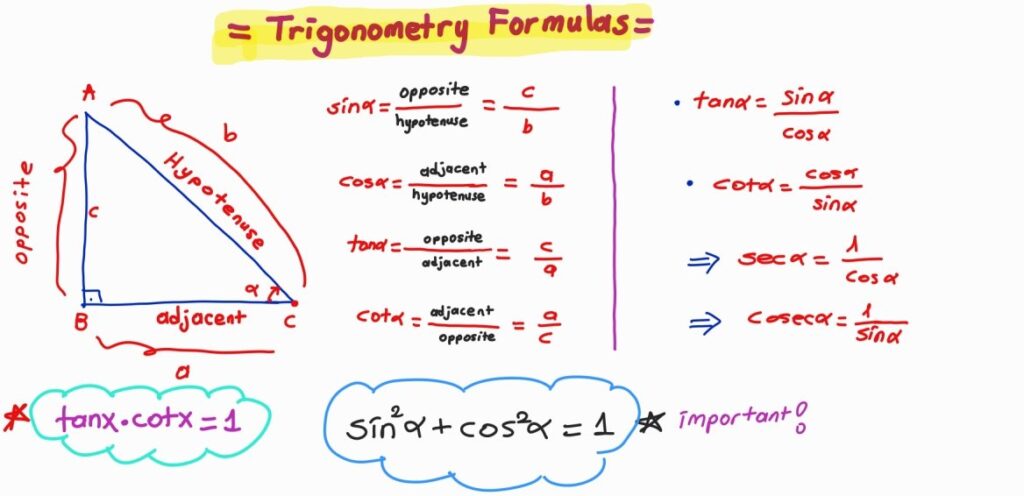
- sin θ = Perpendicular/Hypotenuse
- cos θ = Base/Hypotenuse
- tan θ = Perpendicular/Base
- sec θ = Hypotenuse/Base
- cosec θ = Hypotenuse/Perpendicular
- cot θ = Base/Perpendicular
Trigonometry Formulas Involving Reciprocal Identities
- cosec θ = 1/sin θ
- sec θ = 1/cos θ
- cot θ = 1/tan θ
- sin θ = 1/cosec θ
- cos θ = 1/sec θ
- tan θ = 1/cot θ
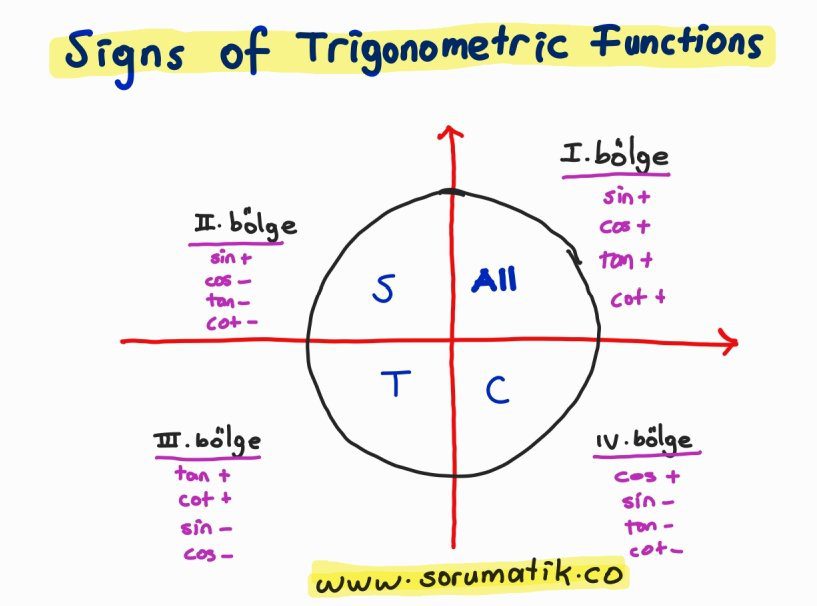
Signs of Trigonometric Functions
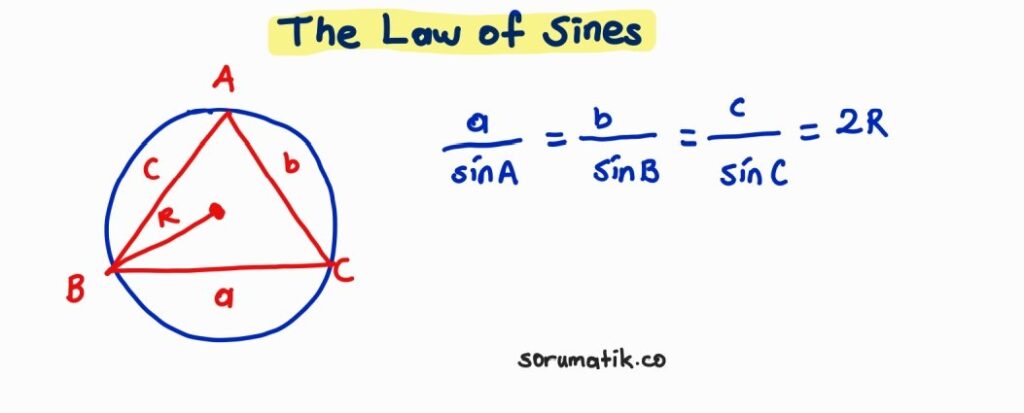
The Law of Sines
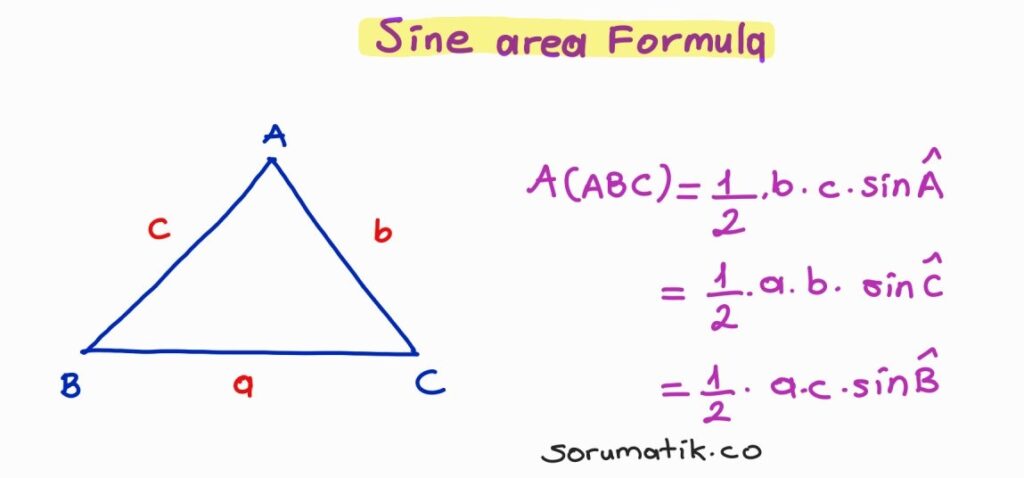
Sine Area Formula
(sin A)/a = (sin B)/b = (sin C)/c
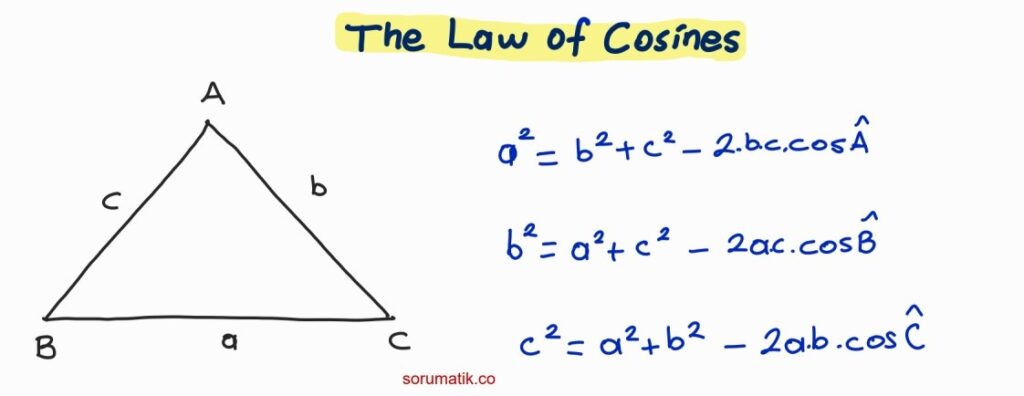
The Law of Cosines
- a2 = b2 + c2 - 2bc cosA
- b2 = a2 + c2 - 2ac cosB
- c2 = a2 + b2 - 2ab cosC
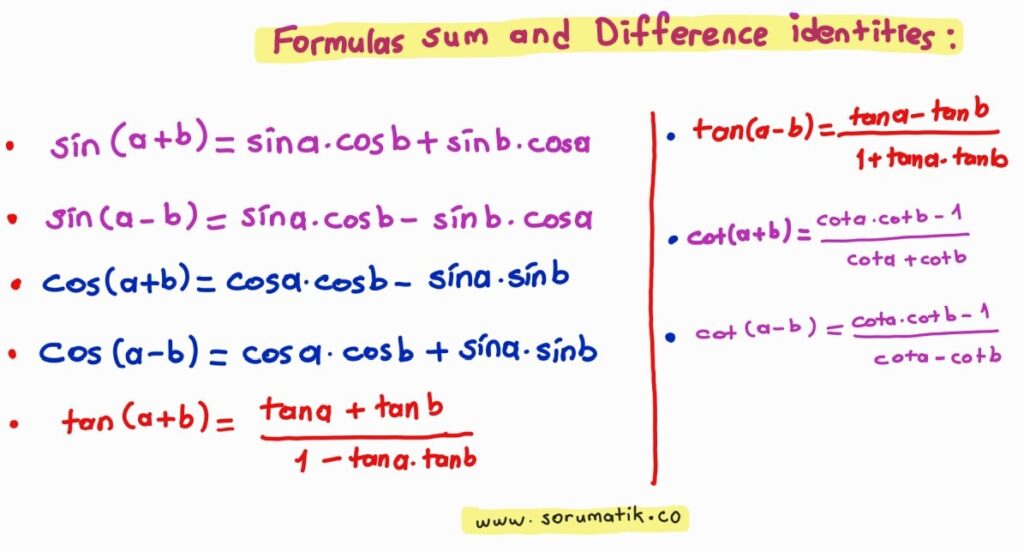
Trigonometry Formulas Involving Sum and Difference Identities
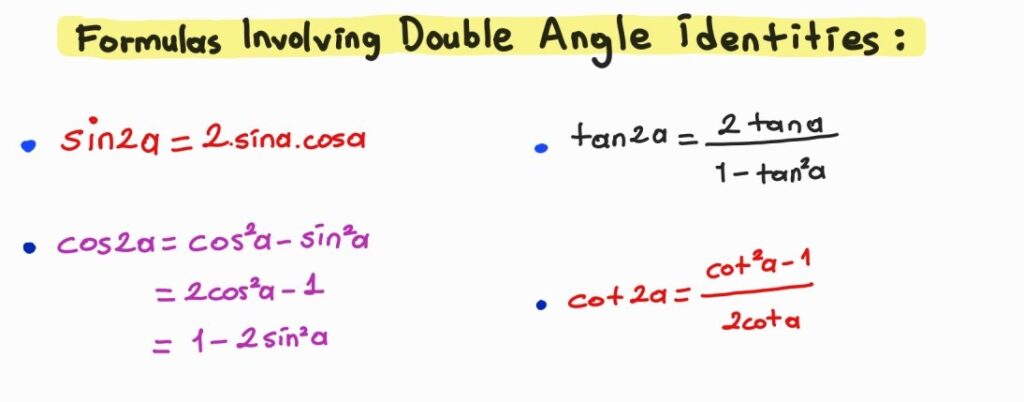
Trigonometry Formulas Involving Double Angle Identities
Trigonometry Formulas Involving Half-Angle Identities
sin (x/2) = ±√[(1 - cos x)/2]
cos (x/2) = ± √[(1 + cos x)/2]
tan (x/2) = ±√[(1 - cos x)/(1 + cos x)]
or, tan (x/2) = ±√[(1 - cos x)(1 - cos x)/(1 + cos x)(1 - cos x)]
tan (x/2) = ±√[(1 - cos x)2/(1 - cos2x)]
⇒ tan (x/2) = (1 - cos x)/sin x
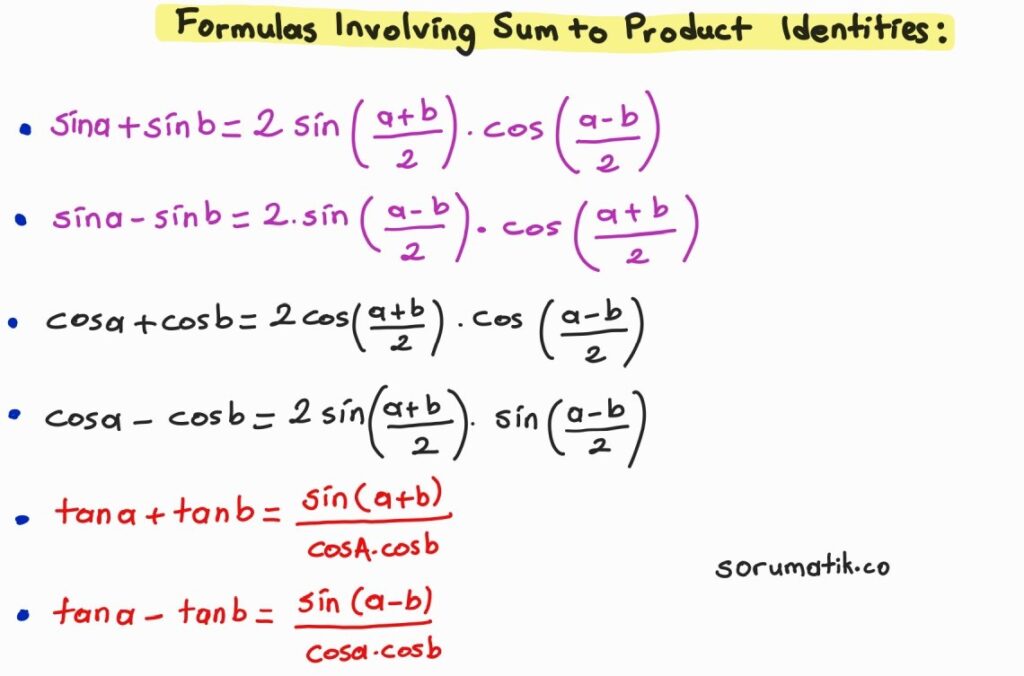
Trigonometry Formulas Involving Sum to Product Identities
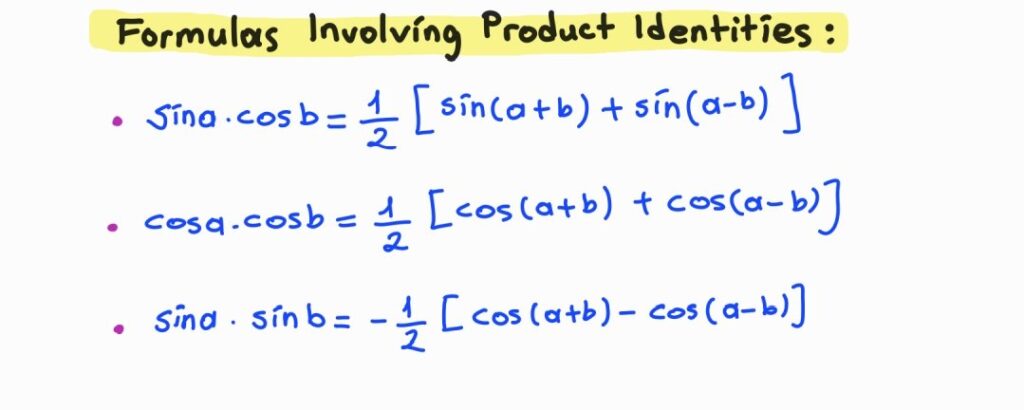
Trigonometry Formulas Involving Product Identities
Trigonometry Formulas Involving Periodic Identities(in Radians)
First Quadrant:
- sin (π/2 – θ) = cos θ
- cos (π/2 – θ) = sin θ
- sin (π/2 + θ) = cos θ
- cos (π/2 + θ) = – sin θ
Second Quadrant:
- sin (3π/2 – θ) = – cos θ
- cos (3π/2 – θ) = – sin θ
- sin (3π/2 + θ) = – cos θ
- cos (3π/2 + θ) = sin θ
Third Quadrant:
- sin (π – θ) = sin θ
- cos (π – θ) = – cos θ
- sin (π + θ) = – sin θ
- cos (π + θ) = – cos θ
Fourth Quadrant:
- sin (2π – θ) = – sin θ
- cos (2π – θ) = cos θ
- sin (2π + θ) = sin θ
- cos (2π + θ) = cos θ
Trigonometry Formulas Involving Co-function Identities(in Degrees)
- sin(90° − x) = cos x
- cos(90° − x) = sin x
- tan(90° − x) = cot x
- cot(90° − x) = tan x
- sec(90° − x) = cosec x
- cosec(90° − x) = sec x
Trigonometry Formulas Involving Reciprocal Identities
- cosec θ = 1/sin θ
- sec θ = 1/cos θ
- cot θ = 1/tan θ
- sin θ = 1/cosec θ
- cos θ = 1/sec θ
- tan θ = 1/cot θ
Inverse Trigonometry Formulas
- sin-1 (-x) = -sin-1 x
- cos-1 (-x) = π - cos-1 x
- tan-1 (-x) = -tan-1 x
- cosec-1 (-x) = -cosec-1 x
- sec-1 (-x) = π - sec-1 x
- cot-1 (-x) = π - cot-1 x
Trigonometry Formulas Involving Triple Angle Identities
- sin 3x = 3sin x - 4sin3x
- cos 3x = 4cos3x - 3cos x
- tan 3x = [3tanx - tan3x]/[1 - 3tan2x]
Bunlar da İlginizi Çekebilir
Tüm math formulas Yazıları →
2027 YKS TYT Konuları ve Soru Dağılımı: Ders Ders Güncel Liste
2027 TYT konularını 10 derste inceleyin; 2018–2026 çıkmış soru dağılımlarıyla çalışma önceliğinizi belirleyin.
![2019-2020 LGS Konuları ve Soru Dağılımları PDF [MEB] 3](https://blog.sorumatik.co/wp-content/uploads/meb-2019-lgs-konulari.jpg)
LGS Konuları ve Soru Dağılımı MEB
M EB LGS konuları ve soru dağılımları bir çok aday tarafından merak ediliyor.MEB tarafından ilk kez uygulandı yapılan sınav da bir çok aday zorlandı.Geçmiş...
YKS 2025 Sayaç - TYT 2025 Nezaman Geri Sayım
YKS sınavı TYT ve AYT olmak üzere iki oturum halinde yapılmaktadır peki yks 2025 Sayaç nedir? TYT 2025 Nezaman? Geri Sayım sayacı ile sizlere bilgi vereceğiz...
![En Çok Tercih Edilen İş imkanı olan En iyi 2 Yıllık Önlisans Bölümleri [ÖSYM-YÖK] 8](https://blog.sorumatik.co/wp-content/uploads/2-yıllık-onlisans-en-iyi-bölümler.png)
En Çok Tercih Edilen 2 Yıllık Bölümler
En Çok Tercih Edilen 2 Yıllık Bölümler ve iş imkanı olan En iyi önlisans Bölümler Y üksek Öğretim Kurumu YÖK en çok tercih edilen en iyi 2 yıllık (önlisans)...
YKS 2026 Sayaç - TYT 2026 Nezaman Geri Sayım
YKS 2026 Sayaç – TYT 2026 Ne Zaman? Geri Sayım Kaç Gün Kaldı? TYT 2026 Ne Zaman Yapılacak? ÖSYM henüz 2026 YKS ve TYT resmi tarihlerini yayınlamadı. Ancak, s...
ÖABT Konuları ve Soru Dağılımları ÖSYM
K PSS ÖABT sınavına hazırlanan adaylar için öğretmenlik alan bilgisi testi ÖABT konuları ' na yazımızdan ulaşabilirsiniz.ÖABT sınavına hazırlanan adaylar KPS...
Yorumları Göster (0) Yorum Yaz / İncele ▾
"List of Trigonometry Formulas PDF" için henüz yorum yapılmamış. İlk yorumu siz yazın!